|
|
This article describes a very simple circuit for single-ended-to-balanced-mode conversion that, belying its simplicity, provides good performance.
I had been intrigued for some time about the possibility of differentially driving a stereo amplifier in order to operate it as a monoblock at twice the single-channel power. As usual, curiosity alone was insufficient motivation. It was the acquisition of a number of 1960s vintage vacuum tube amplifiers along with the lack of a pre-amplifier with balanced output that finally provided the impetus to investigate the possibilities of such an operation along with suitable converters for its achievement.
SYSTEM SETUP
There might be other uses as well. For example, the A75 and DIFF 100 power amplifiers both accept balanced inputs. I have been unable to take advantage of this because I have no preamplifier with balanced output capability. This circuit, placed immediately at the out put of a single-ended preamplifier, could be used with good results if some distance separated the amplifier and preamplifier. In such cases, balanced-line interconnection would take advantage of the balanced amplifier’s common-mode rejection ratio (CMRR) to reduce hum and noise generated over the length of the interconnect run.

PHOTO 1: Eight Citation IIs in one setting.
The aforementioned DIFF 100 amplifier was overly conservatively rated. Using the circuit of this article, the monoblock-operated DIFF 100 delivered 243W into an 8-ohm load at the clipping level, indicating a power level of greater than 120W per channel with both channels simultaneously driven. In general, a pair of similar amplifiers such as the two channels of a stereo amplifier may be driven differentially. Power will be twice that of a single member of the pair.
Thus, for stereo, you could set up a system with the configuration shown in Fig. 1. Each of the amplifiers numbered 1—4 should either be a monoblock or a single channel of a stereo amplifier. Interconnects are standard single-ended RCA types.
Note carefully that the amplifier’s output ground terminals, labeled (—) in Fig. 1, would normally connect to the (—) loudspeaker connectors but are connected instead only to one another in differential-drive mode. Only the amplifier’s “hot” output terminals, labeled (+), are attached to the speakers. The arrangement depicted will provide correct absolute and relative (left channel/right channel) phasing in the stereo setup unless either the preamplifier or amplifier—but not both—inverts the signal.
However, know thy amplifier! For ex ample, the Pass Zen amp not only inverts, but also to maintain correct absolute phase, the output ground and hot terminals were reverse-labeled (+) and (—), respectively, if two Zen amplifier channels are to be converted to a monoblock, you should connect one channel’s (—) terminal to the (+) speaker terminal with the other channel’s (—) terminal connected to the speaker’s (—) terminal, tying the two (+) amplifier out put terminals together in common. The Zen seems to tolerate a shorted output condition rather well, but there are amplifiers whose output would be destroyed by incorrect connections, so be careful.
Since the Zen does invert, you can maintain correct phase by reversing either the connection to the loudspeaker or from the phase splitter. Do not re verse both connections.
BACKGROUND
In the mid-1980s, a former college roommate and longtime friend gave me his old Harman-Kardon (HK) Citation II tube amplifier. The unit was corroded, and the one working channel produced only about 50W instead of its rated 60W. I replaced every capacitor and resistor in the unit, which restored nor mal output in the one working channel. Unfortunately, the second channel’s problem was a defective output transformer (OPT the bane of the tube amplifier owner.
Eight years ago, a new friend gave me one of his two old Citation IIs in return for rebuilding the second unit as a gift for his son. Unlike solid-state amps, you can simply parallel the two channels of many stereo tube amplifiers for use as a monoblock possessing twice the per-channel power capability. The idea of placing two monoblock configured tube amplifiers in my sound system ex cited me. I found a fellow who, at great expense, re wound the defective OPT.
The amplifiers these 120W monoblock-configured tube amps re placed were my 100W pure Class A solid-state monoblocks, whose designs were published in Audio magazine in 1995. I honestly heard no significant difference in the sound between the tube amps and the solid-state amps. However, the possibility of yet another power doubling intrigued me.
A visit to the Audiogon website revealed that a Citation II was available from a musician in Michigan who had at one time used it as a guitar amp. The unit was in a barn in Kentucky, and it was a couple of months before I actually took delivery. It was in reasonably good shape but did have some corrosion as well as a “Dirt Dauber” (sic) nest and spider webs inside. To compensate, the owner threw in a Citation I tube preamp for free.
Soon thereafter, I acquired a fourth Citation II from a seller on eBay. I completely rebuilt both amps, sanding the transformers to bare metal and repainting them satin black. They not only looked mean with black transformers, but, re-tubed with KT-90 output tubes, they would deliver about 65W per channel at 0.2% THD, beating HK’s original specs of 60W per channel at 0.5% THD.
== ==
ABOUT THE AUTHOR
Five time astronaut Norman Thagard was the first American to enter space aboard a Russian rocket for a 90 day mission to the space station Mir With a total of 140 days in space he became the most experienced US astronaut ever In addition to an MS degree in engineering science from Florida State University he holds a doctorate in medicine from the University of Texas Southwestern Medical School He is currently Professor and Associate Dean for College Relations at the FAMU FSU College of Engineering An avid audiophile he designs and builds audio amplifiers as a hobby On May 1, 2004 he was inducted into the Astronaut Hall of Fame located at the Kennedy Space Center Visitors Center Florida.
== ==

FIGURE 1: Single-ended-to-differential conversion in a stereo system.
I simply paralleled the four 16-ohm output taps of two stereo amplifiers to form monoblocks that could deliver 240W into 4-ohm. This was perfect to drive my Martin Logan ReQuests with their 4-ohm nominal impedance, and there was significant improvement in sound reproduction. Since the perception of improved sound persisted even after a year of frequent listening, I was inclined to believe it was real.
If four Citation us sounded so good, what might eight sound like? For one thing, simply continuing to parallel channels would not suffice. Amplifiers are ultimately power supply voltage- limited in their power delivery, and I had “maxed out” with the use of four parallel 16-ohm output taps driving a 4-ohm load.
As I’ve mentioned, you cannot safely parallel solid-state amps, and it is for this same reason that you should not parallel two voltage sources. However, you can safely place voltage sources in series. With amplifiers, you can realize this series arrangement of outputs through differential drive of their inputs, a la the monoblock-configured DIFF 100 bench test. Acquiring and re building five more Citation IIs (you always need a spare), I was in a position to test such a configuration of four stereo amps per channel.
POWER
For initial testing, I used a LF411 op amp in unity-gain inverter configuration to generate the out-of-phase signals required for differential drive. Four channels of two Citation IIs were driven directly by a 1kHz sinusoidal test signal. I ran this test signal through the LF411 inverter to provide differential drive to the four channels of a second pair of amps.
I carried out the initial test with some trepidation. In 1964, I set a speaker on fire by foolishly using it as a dummy load in the engineering lab. While not worried about setting the resistive dummy load on fire in this case, I did have visions of smoke curling from the innards of one or more of the Citation Us on which I had labored, and these power levels were well beyond my previous experience.
I also had never before seen such levels on the AC voltmeter of the distortion analyzer. With the Citation IIs’ 16 ohm output taps in parallel connected to an 8-ohm resistive load, over 58V RMS registered, corresponding to about 425W.
Testing at this level was necessarily brief because the dummy load was a parallel-series arrangement of sixteen 20W noninductive resistors. Specifically to conduct full power, 4-ohm load tests in this configuration, I constructed a second identical 8-ohm, 320W dummy load. In the future, I will have the capability for unrestricted simultaneous testing of both channels of stereo amplifiers into 8-ohm loads at power levels up to 320W/channel or single channel, 4 ohm load testing up to 640W.
I conducted the next test with the two 8-ohm dummy loads in parallel driven by paralleled 8-ohm amplifier output taps. Again, about 425W were delivered at the onset of clipping. With eight 60W amplifiers, the expectation was at least 480W in both tests.
Due to the high output resistance of even large power tubes, most tube amplifiers transformer-couple the output to the load. The Citation II provides three output connections from separate taps on the secondary of the OPT—one each for 4-, 8-, and 16 ohm loads. The rated power of 60W will be delivered only if the load impedance matches the output connection; e.g., if the 4- or 16 ohm tap of the OPT powers an 8-ohm speaker, less than 60W can be supplied.
Was an impedance mismatch the cause of the reduced power output? After all, this configuration was a little more complex than connecting one speaker to one amplifier connection. Perhaps I had failed to account for some nuance of the configuration.
IMPEDANCE MATCHING FOR OPTIMAL POWER DELIVERY
I reasoned that the test configuration was, in simplified form, as shown in Fig. 2. Each voltage generator of magnitude ½Vg represents the output voltage of four paralleled Citation II channels; i.e., all channels of two stereo amplifiers, with Rg = ¼R representing the effective output impedance. Thus, depending upon whether you use the 16-, 8-, or 4-ohm output taps, Rg will effectively be 4-, 2-, or 1-ohm, respectively.
I connected the ground (—) terminals of all eight channels together in common as shown. Note that it is one or an other of the three output taps of four channels in parallel that attach to the top of load R with the second group of four channels attached to the bottom. One group of four channels is driven by a signal that is 180-degree out of phase with the drive signal to the second group, resulting in differential output of magnitude Vg Thus, the already simplified schematic of the left side of Fig. 2 can be modeled in the even simpler form of the right side.
I have omitted any reactance because we are interested in real power and be cause complicating the situation with complex variables adds little to under standing. Although obvious by inspection of the complex power expression, it is offered without proof that if Zg = 2 + ,iKg and = + iX then X = Xg is optimum. Heal power delivered to the load will be:
(1) i [ / (zRg + R )] 2 = v (2Rg + R )_2.
If this were graphed against R with a fixed value for the power would show a peak; i.e., its slope would be zero, at the point R =
To prove this mathematically involves some simple calculus. The slope of this graph is the derivative of the power expression with respect to 14. At a peak or at a minimum, this slope is horizontal and consequently is zero. Thus, you take the derivative, set it to zero, and solve the resulting equation for the optimum value of R:
(2)
The solution to the algebraic equation on the right is R = 2 verifying the graphical implication.
Since in my case I had a speaker whose nominal impedance was 4 and needed to determine which Citation II output tap, 4-, 8-, or 16 ohm to use for maxi mum power delivery, you might think that power should be differentiated with respect to Rg However, there is no value of Rg for which the derivative would be zero because power is maxi mum at Rg =0 and decreases continually as Rg increases from zero. This seems intuitive and can certainly be seen by substituting various values for Rg in the load power expression v +Ri for given fixed values of Vg and Rr Any nonzero value of Rg lowers load voltage (and current) and there fore power delivered to the load.
In any event, the appearance here is deceptive and as you will see, the task really is to vary the (apparent) load resistance to the inherent, fixed genera tor (output) resistance of the amplifiers. Thus, the appropriate action is to solve equation (2).
The typical solid-state audio amplifier has inherently low output resistance even before application of negative feed back. Damping factors of 100 or more with respect to 8-ohm loads can be seen that, given the definition of damping factor, means such an amplifier has Rg < 8/100 = 0.08 ohm Most solid-state amplifiers therefore operate almost like ideal voltage sources, and there is no possibility or need to match impedance. Imagine the current draw and power delivered if the loudspeaker had nominal impedance of 0.1 ohm or less! Of course, there are few solid-state audio amplifiers capable of operating into such impedance.
Unlike a transistorized amplifier, a tube amp has inherently high output resistance, and via its OPT does operate on the basis of matched impedance. Therefore, optimum power delivery should occur when 2 = R = 4 meaning the out put taps used in the present case should be R_tap = 4 Rg = 2R1 = 8-ohm.
REFLECTIONS
While the foregoing discussion seems to imply that by selecting different OPT taps you are changing the amplifier’s output impedance for optimal matching to a fixed load impedance, you are really changing the load impedance seen at the plates of the amplifier’s out put tubes. That impedance is R1 n2, where n is the primary to secondary turns ratio from the utilized secondary tap of the OPT. By changing OPT taps, you seek to select n for which R1 n2 = Rp. Here, Rp is used to represent the output impedance looking into the push-pull output stage of the Citation II amplifier. Rp is typically several kilohms (k-ohm) for a tube-based audio power amplifier.
In summary, I really was varying load impedance for optimal matching to fixed output impedance in line with theory. The test bench results certainly indicated that this analysis applied to the configuration, but why, then, was the power lower than expected?
For all my misgivings about the Validity of my assumptions concerning the configuration and this discussion notwithstanding, I am not such an ex pert on tube amplifiers to state unequivocally that they are completely valid. However, the apparent cause of reduced power output proved more mundane. Finally checking the power line supplying the test bench, I found that the poor 15A circuit supplying the four Citation IIs was sagging badly to 107V AC with the amps driven to clipping.
This is a caution to those who would place very high power amplifiers in their systems. A stock Citation H draws 350W from the power line. Even with the out put stage bias reduced to 67mA/KT-90, my units draw almost 300W for a total of nearly 1.2kW per channel. One wag already jokes about buying stock in the local power company.
It is cold in Tallahassee as I write this, and I rather appreciate the 2.4kW space heater in the sound room. There is a dual outlet on a single 15A circuit and four single outlets on four independent 20A circuits. I had these latter out lets installed by an electrician soon after moving into my current home in anticipation of future folly, so there is no problem with line voltage sag in actual usage.

FIGURE 2: Simplified test configuration.
I ordered a 2kW Variac to replace the old 500W unit on my test bench. This allowed line voltage to the amplifiers to be maintained at 117V AC during full-power testing per Harman Kardon specifications. With this line voltage level, the amps squeezed out 481W at 0.5% THD, exactly what you would expect for eight channels based on single-channel specs.
While waiting for its arrival, I reconsidered the inelegant use of the LF411 inverter as a source of differential drive. There would be more circuitry in the inverted signal path than in the non-inverted path. Although I am a practical engineer with a good measure of skepticism about the audibility of various topologies, types of interconnects, speaker cables, op amps, and the like, I nonetheless aesthetically disliked the asymmetry of the op-amp inverter approach. Was there a simple satisfactory alternative?
THE DIFF AMP
I know well and love the differential amplifier stage. All but one of my amplifier and preamplifier designs have used a dual differential input stage.
The problem is application of feedback in a single-ended to balanced configuration. I played around with a two-stage differential design that yielded pretty good results.
This topology (Fig. 3) has the advantage that no capacitors are in the signal path. The disadvantages are several. For one thing, it requires six transistors, two diodes, and an op amp. Also, although differential output is provided, only the noninverting output is sampled and fed back to the input. With 100% feedback, it is guaranteed that the noninverting output will have a DC level very close to zero, but the symmetry of the circuit is not perfect, so the inverting output will not necessarily have a near-zero DC level.

FIGURE 3: Single-ended to balanced converter using two-stage differential
amplifier.
This problem is minimized by incorporating, a DC servo, implemented with an op-amp-based differencing integrator. At frequencies below [ (1M ohm)(1 uF)]^-1 = 0.16Hz—i.e., at DC—the servo will adjust current source current to the second differential amplifier stage to maintain the inverting output near zero.
Another problem with the circuit of Fig. 1 is that inverting output distortion, while low, was higher than noninverting output distortion. In fact, noninverting output distortion was below the 0.003% THD floor of my Krohn-Hite distortion analyzer.
Were I to use the circuit, I would choose different transistors. The matched dual n-channel JFET is no longer available, and the TIP 30 pnp BJT is commonly used in power applications. Even so, while the devices and values shown worked well enough, was there a simpler solution?
THE CONCERTINA PHASE SPLITTER
When tube amplifiers ruled the audio world, it was necessary to provide differential drive to the push-pull output stage. This is not a requirement in complementary-symmetry push-pull output stages, but tubes do not come in complementary variants.
Not surprisingly, differential amplifier stages were often used to generate the two out-of-phase drive signals required for push-pull operation. There was an alternative that was also widely used because it offered good performance in a simple topology. It was called the concertina phase splitter. The tube-based version is shown in Fig 4.

FIGURE 4: Concertina phase splitter.
Obviously, this is simplicity itself. Rp was made equal to Rk, so neglecting any load effects and assuming grid current is zero, plate current, i must equal cathode current, ‘k’ and both output voltages, —i and ip Rp, must have equal magnitude. Gain magnitude is, in fact, unity, which is readily understood, given that the non-inverted output is that of a cathode follower. The phase splitter is, in fact, both a cathode follower (common-plate) and common-cathode amplifier.
Exactly analogous to the behavior of common-emitter or common-source transistor stages, the output taken at the plate (common-cathode) is inverted from the input because increased input voltage produces increased plate cur rent, greater voltage drop across and consequently lower voltage at the plate. The circuit therefore does its job of accepting a single-ended input and generating differential outputs at unity gain.
Not shown in Fig. 4 are the two or three coupling capacitors required by the circuit. After all, neither the grid nor either of the two outputs is at ground potential. At the input, you need only a small capacitor because the grid resistance is so high. At the output, however, the input resistance of the thing being driven will dictate the necessary minimum capacitor value.
THE TRANSISTORIZED SOLUTION
The transistorized phase splitter circuit is shown in Fig 5. The coupling capacitors should be large enough in value that response is flat down to 20Hz. For that to be the case, the cutoff frequency should be at least one decade below 20Hz or 2Hz.
For my case, each output drives all four channels of two Citation II amplifiers. The Citation II has an input resistance of 1M ohm, the phase splitter outputs see 250k-ohm, and a capacitor of 1uF is sufficient for a low-frequency cutoff f = (2 pi RC)^-1 < 1Hz. Conservatively, two 2uF film capacitors in parallel formed the output coupling capacitors.
I employed little conservatism at the input. There, the resistance posed to the driving source is R_in = RB || [Beta (RE + r_e)] where R_B = R1 || R2 = 15k || 3.29K = 10.8k and RE = 470-ohm is the extrinsic emitter resistor. The intrinsic emitter resistance— the resistance “seen” looking into the emitter—is re = V 25mV/25mA = 1 ohm. This expression is derived from the exponential Ebers-Moll equation that models the transistor’s transconductance behavior.
Quiescent collector current, I_CQ, for this circuit will be seen to be nearly 25mA, hence the use of that convenient value in calculating re. For almost any value of extrinsic emitter resistor, it is apparent that re can be neglected, especially if I_CQ is more than a few milliamperes.
I purchased the particular 2SC2682 BJTs that I placed in the two-channel phase splitter several years ago from a lot whose devices were guaranteed to have Beta >= 280. Therefore, circuit input resistance is expected to be around 10k-ohm, which was the desired design value to avoid loading the preamplifier. The 10uF capacitor specified results in a cutoff frequency slightly less than 2Hz. With physically small but otherwise good-quality 5uF film capacitors in my parts bin, two in parallel couple the pre amplifier signal to the phase splitter. Response at 20Hz was less than 0.1dB down from the 1kHz response, based on the 0.1dB resolution of the AC volt meter in the distortion analyzer.
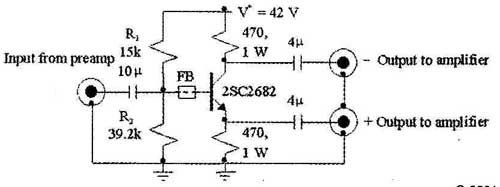
FIGURE 5: Bipolar junction transistor phase splitter.

FIGURE 6: MOSFET phase splitter.
It is possible to eliminate the input coupling capacitor through the use of a MOSFET, as shown in Fig 6. You could attempt this scheme with a BJT, but you would need to eliminate the 51.1k-Ohm ground reference resistor at the input. This would make the circuit ground reference depend upon the signal source, leading to a long circuitous ground path, which is probably not a good idea.
Then, too, any interruption in this path, such as can occur when the pre amplifier is switched from CD to tuner, may momentarily lift the ground reference. This will upset the bias of the BJT, potentially producing a loud “pop.” I know from trying this, but the scheme worked quite well as long as the input was unchanged. However, with eight 120W tube amplifiers waiting to exercise all 960W of their capability, pops were disallowed.
The advantage of eliminating the input coupling capacitor is obvious, be cause good-quality 10uF film capacitors are neither cheap nor physically small. One resistor is also eliminated.
The MOSFET-based splitter had significantly higher distortion than the BJT version. On the plus side, this distortion was nearly constant at about 0.02% over the entire 20 - 20kHz spectrum.
Another disadvantage is that a bipolar power supply is required. Quiescent source voltage will be about 3.5V, so a negative supply of i results in 11.5V across the 470 ohm source resistor. This is very close to the desired value of ¼(V+ - V-) = ¼ [30 - (-15)] = 11.25V, which leads into the discussion of bias.
BIAS LEVEL
It is a simple matter to determine the proper bias conditions for the phase splitter. The extrema occur when the active device—be it MOSFET, BJT, JFET, or vacuum tube—is fully off or fully on. If fully on, both outputs -- (neglecting BJT saturation voltage) will be -½(V+ - V-). If fully off, inverting output will be at V and noninverting output will be at V-.
For symmetrical and therefore maxi mal undistorted output voltage swing, source or emitter voltage should be at ¼(V+ — V-), while drain or collector voltage should be at ¼ (V+ - V-). This al lows both outputs to swing as much as ±%(V — V) about their quiescent states. For simplification in the single supply case, note that V =0.
To keep distortion reasonably low, you should limit swing to slightly less than ±¼(V — Vi). A kind of standard sensitivity for audio power amplifiers is to produce full-power output at about 1V ELMS input or so. The Citation II is designed to deliver 60W per channel into 4-, 8-, or 16 ohm loads when input is 1.5V RMS.
There are amplifiers with significantly lower sensitivity. Again using Nelson Pass’ Zen amplifier as an example, a source capable of 3.5V is said to be the requirement. The beauty of the concertina phase splitter is that almost any sensitivity can be accommodated if sup ply voltage(s) is raised sufficiently. A note of caution: choose devices whose V_CE_max rating is not exceeded.
I desired some overload margin, primarily for design conservatism. A 42V power supply is sufficient for output voltage swings up to 21Vp-p, with good linearity up to 18Vp-p or so. This translates to 6V RMS for 12dB overload mar gin relative to 1.5V RMS. With 1V RMS output at 1kHz, the as-constructed amplifier had 0.003% THD at the noninverting and 0.005% THD at the inverting output. This increased little until 3V RMS was exceeded, reaching slightly less than 0.05% at 5V RMS. Again, you can control this almost at will by use of progressively higher supply voltage(s).
As for the choice of load resistors, I chose the value on the basis of the minimum that would not require transistor heatsinking. Even small-signal transistors can usually tolerate 1/4W dissipation. With the 42V power supply, V_CEQ = 20V. ICQ = I_E = 1/4(V+ - V-)/R_E = 1/4(42 — 0)/470 ohm = 23mA, and transistor power dissipation is about 20 x 0.023 = 0.46W. The 2SC2682 is a medium-power transistor that can easily dissipate 1/2W without a heatsink.
A minimum value for load resistors is desired because inverting output resistance is essentially equal to the collector load resistor value, and keeping output resistance small minimizes loading effects. To drive four paralleled Citation II channels such small values are unnecessary, but amplifier input resistances can be as low as 10k-ohm or even lower and a 1:10 ratio is usually recommended. Therefore, to give this design more general utility, I applied this criteria in calculating load resistor values.
STABILITY RULE OF THUMB
Refer to Fig. 5 to calculate base bias resistor values. The requirements were Rm=R 10k-ohm VB= VE + V_BES = ¼(V+ - V-) +0.7= 11.2V. To en sure that adequate base bias current would be available, I also applied the stability rule of thumb, R_B =0.1 Beta R_E
A strict calculation of the divider ratio required to simultaneously ensure a value for RB such that R_in = 10k and VB = 11.2V involves solving Kirchhoff’s voltage law around the Thevenin-equivalent base-emitter circuit, which sounds worse than it actually is. The rule-of-thumb ensures the “goodness” of the voltage divider so that, to the accuracy that is needed, you can assume that currents through both resistors comprising the divider are equal.
The numerical conditions based on this are [ + R — V-) = 1L2V and RB { + re)]Rin}I + re) — R where = 10k-ohm. The former equation ensures that R1 and R2 will be such that emitter voltage will be ¼V+ i.e., VE= VB— VBEE 11.2—0.7= 10.5V= ¼V+. Since collector voltage will then necessarily be about ¾V = 31.5V, you can achieve maximum symmetrical voltage swing at both outputs. The latter equation constrains R and R to values that, in conjunction with RE = 47-ohm will maintain input resistance at or greater than 10kl per the design criteria. Of course, there is also the rule of thumb RB = 0.1 Beta R_E.
There are three conditions, but only two unknowns. While this might suggest that the values of R1 and R2 are over-determined, this is not the case. The rule of thumb is potentially in conflict with the input resistance criteria. If this sounds confusing, consider the actual design sequence:
1. Set power supply voltage to allow 5V RMS output.
2. Set collector load resistor to the lowest value consistent with no heatsink operation; i.e., to 470-ohm and let RE = RC.
3. Choose RB consistent with emitter voltage VE = ¼V+ = 10.5V, and R_in >= 10k-Ohm.
4. Verify that R_B = 0.1 Beta R_E
The tendency will be for the rule of thumb to require values for R1 and R2 that are too low to meet the input resistance criteria. If, in step 4, RB>> 0.1 Beta R_E, then a possible solution is to choose a transistor with higher cur rent gain. With Beta = 280, the 2SC2682 is already close to the highest available in a medium power transistor, but I tried the MPSA18 with Beta >= 500 at the breadboard stage and it worked OK with slightly higher distortion than the chosen device, so it could be a suitable alternative.
On the basis of these equations, numerically for this design R2/(R1 + R2) = 0.27 and R2/(R1 + R2) >= 10.8k-ohm. This leads immediately to R1 10.8k-ohm or R >= 40k-ohm. The closest standard 1% value is 40.2k-ohm but I used 39.2k-ohm because I had several 0.5% metal film resistors of this value in my parts bin. I accepted the fact that input resistance might be slightly less than the desired design value.
R2 = 0.27R1 — 0.27R2 = 0.27R — 0.27) = 0.37R = 0.37(40k) = 14.8k-ohm
The closest 1% value is 14.7k-ohm but I used 15k-ohm resistors because I already had them on hand. Input resistance with the schematic values should be at least 10,023 ohm so the input resistance criterion is met despite the deviations from the calculated values. Emitter and collector voltages differ a little from ideal due to the resistor values, which slightly reduces the overload margin but otherwise is of little significance.
Checking the rule of thumb, ….This indicates that input resistance could have been raised a bit without violating the rule-of-thumb. After all, the rule-of-thumb is an approximation, not a rigidly fixed value, so the energetic builder might choose to raise and R values to reach an input resistance of 15k-ohm
PERFORMANCE SUMMARY
I’ve already partially covered the circuit performance parameters in the text. To summarize, at 1V RMS output, THD was 0.003% at 1kHz for the noninverting and 0.005% at the inverting outputs, rising to 0.006% and 0.009%, respectively, at 20kHz. Response was flat within 0.1dB from 20 -20kHz. These results were identical for both channels that were constructed.
Matching between inverting and noninverting output levels was 0.1dB, even though emitter and collector resistors were only 2%-tolerance metal film power resistors, which were not selected but were from the same DigiKey lot. By placing higher-value resistors in parallel with either the emitter or the collector resistor as appropriate, you could achieve any degree of de sired matching.
With the 2SC2682 BJT, the circuit oscillated unless power supply bypassing was carefully attended. The ferrite bead shown in the BJT circuit eliminated this oscillation without regard to power-sup ply considerations. I saw no oscillation with the MPSA18 transistor. The gate resistor for the MOSFET circuit is recommended for the same reason the ferrite bead was employed in the BJT circuit. It may seem strange that a unity-gain circuit can oscillate, but it can.
For the lawyers among you, let me state that I assume no responsibility for damage to amplifiers or humans in the use of the phase splitter. I bench-tested the variants shown, and the recommended version has been in the 480W per channel system for six months with no problems as of this writing. However, it was pointed out that amplifiers differ in their internal configurations and if not taken into ac count, this can lead to improper inter-connections that can cause amplifier damage. Inadvertent failure to connect inputs to one Citation II resulted in a dull red glow of the KT-90 plates at high volume as the working amplifier at tempted to drive the idle amplifier’s outputs. Fortunately, this was quickly corrected with no damage.
This article describes one circuit. Two are required for stereo as shown in Fig. 1. All parts including the specified transistor should be readily available. I found an Internet source for the 2SC2682 at a unit price of $0.97. You can use many transistors as substitutes. The SK9041 and NTE373 are replacement series devices roughly equivalent to the 2502682.
Of all the transistors I used, the 2502682 provided the lowest distortion, but did not beat the MPSA18 by much in this regard. The MPSA18 has the ad vantage that typical current gains are 1,000, permitting the input resistance of the circuit to be raised to nearly 50k-ohm. On the other hand, the MPSA18 is not usually operated at 23mA collector current, although this does not exceed Motorola’s rating.
FINAL COMMENTS
I guess that there are those who would like to read some comment about the 480W per channel, tube amplifier performance. It has been my pleasure to listen to some pretty good systems, including the one at Pass Labs used for auditioning some of the finest solid- state preamplifiers and amplifiers to be had.
First, the system includes a TNT 3.5 turntable, JMW 10 tonearm, and Audio Technica OC9ML/II moving-coil (0.4mV) cartridge. The phono pre-pre amplifier is a version of a design published earlier and modified for greater gain to accommodate the low-output cartridge. The output of the phono pre-preamp directly feeds the input of a Krell PAM-S preamplifier, which feeds the phase splitter, which, in turn, drives the eight Harman Kardon Citation II tube amps in a four and four, 480W per channel arrangement for stereo. Loudspeakers are Martin Logan ReQuest hybrids. For SACD/ CD/DVD source material, I used a Philips 963 player.
There is no question in my mind that power and lots of it is required for accurate reproduction of sound. Even for those with very efficient speakers, I believe the sound would benefit from higher-powered amplifiers. Power- handling capability of the ReQuest is 250W and few loudspeakers can handle 480W, even on a short-term basis.
Obviously, the idea is not to achieve speaker-damaging power levels. A circuit operating closer to its small-signal ideal is inherently more linear, and I believe that this at least contributes to the improved sound. Then, too, sound sensitivity is logarithmic, so squeezing out those last few dBs of sound pres sure level at loud volume is not possible without power reserve.
There is—I have heard—a tendency to turn up the volume to just below the point where distortion becomes objectionable. This notion certainly coincides with my experience. For realism, it seems that levels must be close to live levels. Based on sound-pressure levels, the volume is now significantly higher, but it paradoxically sounds no louder than before. Some of you may have wondered why a live orchestra sounds good, while the reproduction of an orchestral performance in a sound system can sound unpleasantly loud even though the actual sound pressure levels are less in playback than in performance.
THE BIG LEAGUE
With the current system, the effect is almost visceral. Joy knows no bound when the reproduced sound of an instrument is suddenly so real that it evokes involuntary laughter. Every aspect of the listening experience that I can think of is better now, and I believe that I have moved into the big league of sound reproduction.
I saw an Internet ad for a used 90-tube, 900W per channel amplifier recently. The price was in excess of $60,000. Although the assemblage of Citation us described here has only about half that power rating, the total cost including replacement of every resistor, capacitor, and tube was less than $8,000 spread over many years. With good, used tube amplifiers often advertised on eBay and Audiogon, this approach is a relatively inexpensive way to join the big league.
Before motivating you to do some thing foolish, I should point out that this method of power augmentation is not for the faint-of-heart. The amplifiers occupy a lot of real estate, weigh a total of 500 lb, and the idle power consumption makes the amplifiers expensive to operate, a factor exacerbated by a requirement for increased air conditioning in warm weather.
Interconnect cost can be enormous if you purchase audiophile cables. Because I placed the phase splitter in an old SQ quad converter (how appropriate) utilizing the ten RCA 1-m stereo interconnects. Although I used some inexpensive (but nonetheless Stereophile-recommended) AR interconnects at about $20 each, total cost came to nearly $300. Similarly, there are 18 speaker cables to manage. For this purpose I built two adapter boxes, one for each channel.
I beg audiophiles’ forgiveness because 1’ lengths of 18-gauge zip cord connect the Citation output terminals to the adapter box. Internally these are connected appropriately to two heavy-duty, five-way binding posts. Connection from these binding posts to the terminals on the ReQuests is via 12-gauge Monster Cable terminated in dual banana plugs. You must take great care in the management of so many connections.
On the input side, some of the complexity would be removed by using a balanced preamplifier, although I would still have to construct XLR-to-RCA adapters. Even so, at some point, I in tend to replace the phase splitter and the Krell with a balanced preamplifier. For now, I’m too busy listening.
I would like to thank Mr. Jim Mc Shane for his useful information as well as parts for Citation II restoration.
REFERENCES
1 Norman Thagard and Nelson Pass Build the A75 Power Amplifier Parts 1 & 2 TAA 4/92 & 1/93
2 Norman Thagard A 100W/Channel AS Differential Input Amplifier Parts 1 & 2 audioXpress Nov and Dec 02
3 Thomas C Hayes and Paul Horowitz; Student Manual for The Art of Electronics; p 221 Cambridge University Press 1989.
4 Norman E Thagard A Phono Pre-Preamplifier for the CD Era (audioXpress Jan and Feb 01)
============