By Sam Wilson, CET
Some time ago I wrote about the models that are used for teaching about capacitors. It is necessary to resort to models in order to give beginning students an idea about capacitors that they can visualize.
As far as I'm concerned, the hardest course to teach is beginning electronics. You have to start everywhere at once. It is necessary to get the beginning student into the subject even though that student may have no background knowledge. Models make it possible to get the student into the subject in a short period of time.
There is the danger that students learning by models will get married to them. That gets in the way of their learning more advanced concepts as they come to the point where the model doesn't work. It can easily be avoided by letting the students know they are being given a concept that will have to be modified as they advance in their studies.
This month I'm going to start by reviewing the procedure for charging a capacitor when there is nothing connected to its leads.
This procedure doesn't fit with the model that is usually used for teaching capacitors. I'm also going to talk about the voltage across resistors that have nothing connected to their leads. As with the capacitor discussion, this doesn't fit the model usually used for resistors. However, that voltage is very important for making certain types of measurements.
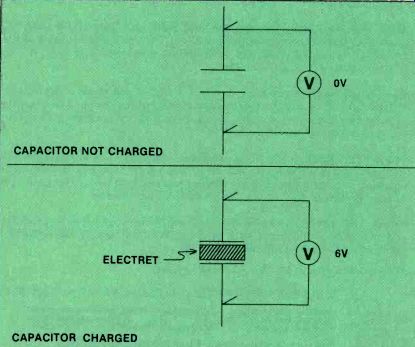
CAPACITOR NOT CHARGED CAPACITOR CHARGED
Figure 1
Charging capacitors without using their leads
A popular way of teaching about charging and discharging capacitors is actually a model for that operation. Students are told that capacitors become charged by forcing electrons into one plate of the capacitor and sucking them out of the other plate. The capacitor is supposed to be charged when the excess electrons are trapped on the negative plate and electrons are prevented from getting into the other plate.
That concept of charging a capacitor disregards the very important role played by the dielectric.
As a matter of fact, a capacitor can be charged and discharged without using its leads, but not without using its dielectric. (See Figure 1.) An electret is a permanently charged dielectric. At one time electrets were nothing more than a lab curiosity. Today they are used in speakers, microphones and other electronic devices. When used as a permanent (read-only) memory, they are guaranteed to hold their charge for 99 years.
You can make a simple electret by placing melted wax between two large plates that form a capacitor. Connect a very large voltage across the capacitor and keep it there while the wax cools and hardens. The wax will be an electret. (This experiment was described in a previous issue.) The voltmeter in Figure 1 shows that there is no voltage across the capacitor at the start of the experiment. Placing the electret between the capacitor plates produces a voltmeter reading, showing that the capacitor is charged.
The idea of that experiment is to show that the dielectric actually stores the energy in the capacitor.
Before discussing the voltage across resistors that have no connections to their leads, it would be a good idea to take a fantastic voyage.
A semiconductor trip
I once saw a movie titled "Fantastic Voyage" (or something like that). People were put into a space ship and reduced in size. Then they were injected into the veins of a man. As I remember, they had to make their way to his brain. The plot may not be too solid, but the movie was a complete course in the operation of a human as a system.
We're going to borrow that space ship and travel inside a semiconductor material. That way, we can see for ourselves what Brownian motion and intrinsic currents are all about. It will help to review these things before we talk about that voltage across resistors.
We're going inside a small block of germanium, but we would see the same thing in other semiconductor materials.

Figure 2
This time we will just journey to a point that is barely inside the surface. In the next issue, we will travel through the material and stop at such tourist attractions as grain boundaries, interstitial atoms and other deathnium traps.
Some of the people who are with us are not prepared for the first inside view of the material. They are not technically trained people. The geometric arrangement of the atoms is perfect and orderly. It looks like some kind of man-made work of modern art.
Every atom is perfectly positioned, and each atom is in a violent vibrating motion. We explain to the non-technical passengers that this is called Brownian motion. We would see this regardless of the type of material we had entered. At a special command, the people outside apply a small increase in heat and we see the motion increase.
Occasionally we see a small particle thrown off a vibrating atom.
It looks like a tiny space ship as it moves between the atoms and lands at a distant atom. Those particles are electrons, and their motion through the material is actually a form of electric current, called intrinsic current.
When the temperature of the material is increased, the number of electrons traveling between atoms is also increased. It is this motion of electrons that we came to see, so we end the journey.
Noise voltage You know that at room temperature there are electrons that gain enough energy to escape from an atom or molecule. The motion of those electrons--called intrinsic current--is in random directions.
Except for the few electrons moving at right angles to the resistor axis, the motion of electrons can be resolved into two vector motions. This is shown in Figure 2.
One is the horizontal vector, which is of no interest here. The other is the vector in the direction of the leads.
At any one instant in time there will be more vector motions toward one terminal than toward the other terminal. That terminal is very slightly negative with respect to the other terminal for the instant in time being considered.
During the next instant in time there will be more vector motions in the opposite direction and the voltage across the resistor will reverse.
The overall result is that there is a very small amount of ac voltage across the resistor when it is lying on a bench with nothing connected to its terminals. That is called the noise voltage of the resistor. The noise is called Johnson noise or thermal agitation noise (sometimes just thermal noise). If you like your theory laced with a little bit of math, the noise voltage can be calculated from the equation:
V , a, = NI4kTAfR where k is Boltzman's constant (I'll discuss this later), T is the temperature in Kelvin (that is, degrees C + 273°), Af is the band of frequencies of interest, and R is the resistance of the resistor.
Applications
How does this affect the everyday work of a technician? Well, you can't do anything to change Boltzman's constant or the number four. However, you can see the advantage of cooling resistors in noise-sensitive circuits.
Also, the bandwidth of the circuit involved affects the noise, so it is important not to have a wider bandwidth than is needed for the job. Remember, noise and bandwidth are tradeoffs.
Actually, the noise equation doesn't tell the whole story. It is for use with copper wire. (It is a long time between times when we see copper-wire resistors.) For practical resistors the amount of noise is greater than indicated by the equation. Table 1 shows how practical resistors compare.
Table 1.
Resistors and noise
Type of Noise resistor problem carbon worst film better wirewound best Use of noise in evaluating amplifiers When you connect a resistance across the input terminals of an amplifier, you have injected noise into that amplifier. For example, connecting an antenna to the RF amplifier of a TV receiver injects noise because of the antenna resistance. As another example, a resistive transducer (such as a photoresistor) connected to an amplifier injects noise.
The semiconductor amplifying devices (bipolar transistors, JFETs and MOSFETs) are made with semiconductor materials, so they produce Johnson noise.
Manufacturers sometimes give a noise rating for a device or for amplifiers, stating its equivalent noise resistance. That is the resistance of an imaginary resistor that produces the same amount of noise as the device (or amplifier) being rated. A low value of equivalent noise resistance is preferred in most cases.
Equivalent noise temperature is another way of evaluating noise. It makes use of the noise power equation for resistance: P a> = kTAf where k, T and Af have the same meanings as in the equation for noise voltage.
Also see: The meter said ... what?