|
|
Previously, we identified noise as a problem to good sound and examined ways in which we could control it. The second impediment to good listening or speech recognition is the self-masking of the sound by itself. Before the advent of modern acoustics, this was sometimes called resonance, or reverb. In today's politically correct atmosphere, you need to be careful about what you call things.
An echo is a single clean reflection of a sound. Reverb is a stream of multiple echoes, decaying into an indistinguishable muddle of noise. Resonance is a single frequency or band of frequencies that decay at a very slow rate. If the energy being fed into the resonance is greater than the decay, this results in a tone. A canyon echoes, a room reverberates, an organ pipe resonates.

PHOTO 1: Echo Busters
ACOUSTICS LESSON
The prediction of decay in a room was the first major step in the modern practice of acoustics. This started at Harvard University where Wallace Clements Sabine, a junior faculty member, was asked to investigate why a new lecture hail was not performing as expected.
He started with a pair of lecture halls, an assortment of organ pipes (C"), a stopwatch, compressed air, and seat cushions. He sounded a pipe until the volume in the room became very close to steady-state, then he turned it off and timed how long the sound took to decay.
He tried single organ pipes and multiple pipes of the same tuning for increased amplitude in a variety of rooms.
Sabine devised a formula for predicting the decay of sound based upon the volume of a room and the total absorption in it. He found that the decay of sound increased as he added absorption.
It did not seem to vary with increases in starting level. It took a bit of time for the sound energy to rise to an almost steady level. Using a decay ratio of 1,000,000 to 1, he came up with this classic equation:
Time to decay 1,000,000/1 = .16 x Room Volume/Total Absorption.
For those in the know, that would be right! He used the metric system of measurements.
In some early experiments Sabine instructed a panel of experts to listen to a pianist while he added seat cushions to the rooms. All the rooms were furnished differently and varied in size. By adjusting the added absorption, Sabine was able to determine that the preferred reverb time occurred at just about one second. He was forced to measure the reverb time with organ pipes and a stop watch at night when no one else was present and it was quiet enough.
These and other experiments make it clear that Sabine changed the science of observing the behavior of sound into the engineering practice of using knowledge to predict and design. He published his first work in the periodical The Amen can Architect (1900).
VOLUME
There were a few changes that followed in this country. The English system of measurements changed the formula to T = .05 x V/A.
Absorption is now measured in sabins. One sabin of absorption requires that all the sound is absorbed in an area of 1ft^2 (or meter) Zero sabins means no sound is absorbed, it is all reflected. A ratio of 1,000,000 to 1 is now referred to as 60dB.
Sabine understood that it takes time for the energy to build up in a room and then decay. He determined that decay was based on the absorption and geometry of the room and knew that as more people entered the room they absorbed some of the energy. He was the first to define the difference between re verb and resonance and established that under normal conditions reverb time is not level-dependent. He even deter mined that the optimum reverb time for a given room was volume-dependent. He suggested 1 second for 10,000ft^3 and 2 seconds for 1,000,000ft^3 In a large room the extra reflected sound helps to naturally amplify speech and music.
Sometimes you need to calculate the reverb time for a room before it is built or renovated. This is reasonably easy to do. Remember the reverb time for many rooms is .05 x volume/absorption. You must be able to calculate the volume of the room and the total absorption. For a rectangular room the volume, of course, is the height x the width x the length.
ABSORPTION
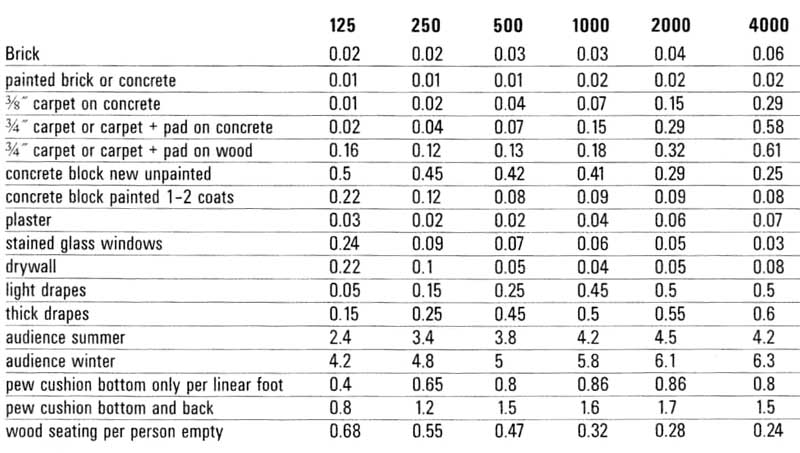
To calculate the absorption, note what the walls, floor, and ceiling are made of. Then consult a chart to find out the absorption of that material. The chart will show the absorption for different frequency ranges. If you are just considering speech, use the 2000Hz data only. Sabine used C”, about 512Hz; we still use 500Hz for music rooms. If you are careful, prepare a calculation for each frequency.
The values for absorption originally were determined by applying sound energy perpendicular to the material and measuring the absorption. But the bad news is that sound in a room does not always hit dead on. That is why the currently accepted method is to place much of the material in a reverb chamber and measure how it decreases the reverb time, although this gives a lower value because not all of the energy hits dead on. The error here is that the average angle in a reverb chamber is still greater than the average angle in a large room. It is just about right for listening rooms or classrooms but frequently high for cathedrals.
If you are considering cathedrals or stadia, a more accurate method is to use the highest absorption value and then calculate the average angle at which the sound strikes the material. Then multi ply the cosine of this angle squared by the highest absorption value to get the value for that angle.
Another phenomenon is that the same amount of absorption spread out over a room will reduce the reverb time more than if it is all clumped together. Some of this may be due to higher frequencies propagating as waves, where as the lower frequencies inside a room do not have enough length to form a wave so they behave as pressure modulations. The higher frequency waves hit ting the spread-out material may have some phase shift induced by the slower propagation near the absorbent material, resulting in additional energy loss. The lower frequency pressure modulations are perhaps guided by the untreated area into the absorption so the increase in edge area increases the total absorption.
AIR COUNTS
Table 1 is a chart of typical absorption values, which differs from the numbers you may find other places. I started with other folks’ numbers and sent my people to measure larger rooms and compare the predictions with the measured results. I kept notes, and this table is the result.
Take the floor, for example. If it is carpet, determine the thickness, pick the value, and multiply that by the area of the carpet. That is the absorption for the carpet in the room. If parts of the floor are concrete, use the value(s) for concrete times the area. Concrete block is a common building material with a giant problem!
When I attended college, the reason ably new recitation rooms were concrete block rooms with a concrete floor and ceiling. There were no windows, because electricity was considered reliable enough to provide all the light required. The concrete block walls and student bodies provided enough absorption so that speech intelligibility was good.
In those ancient days material was presented with chalk on a real black board! The problem was that the erasers for the blackboards would fill with chalk dust and thus needed to be cleaned. In a classroom without windows the solution was to pound the erasers against the wall leaving white marks. Eventually the walls needed to be painted, thus lowering the sound absorption in the rooms to almost zero! Speech intelligibility dropped to awful. Now the fix was to add acoustic ceilings, but to save money, they used cheap but more durable tiles. There was just enough absorption to bring the rooms up to adequate.
The moral of this tale is that when designing with concrete block as the absorption material, sooner or later it will be painted.
Be sure that you total all of the surface areas with their values. Add to this total of the boundary absorption your best guess for the padded furniture and people in the room. This grand total is the number you use in the equation. In big rooms air counts!
A quicker, simpler method is to assume everything absorbs zero except for carpet, furniture, drapes, and other fuzzy stuff—these have a value of one. Your absorption value will be the surface area of all your fuzzy stuff.
Once you have the volume and absorption for the room, calculate:
.05 x Volume/Absorption
The number should be more than zero and less than ten.
The biggest problem with the estimate is that the Sabine equation predicts a reverb time even for a room in which everything is absorbent. The equation works for most rooms, but probably not for your listening room.
BOUNCIN’ ROUND THE ROOM
The method for calculating the reverb time of rooms that are not very live is the Norris-Eyring equation. For the Sabine equation you needed to know the volume of the room and the total absorption.
Now you need to know the surface area of the interior. This is 2 x the length x width + 2 x height x width + 2 x height length for rectangular rooms.
You now must calculate the average absorption per square foot, by dividing the total absorption value by the newly calculated interior surface area. This number should be between zero and one. Subtract this average absorption number from one. Again, this should be between zero and one.
Here comes the hard part: Get a scientific calculator that has a key marked “LN.” Enter your 1-A number and press the “LN” key. You should now have a number between zero and -10. In theory the number could go to negative infinity, but your calculator will tell you “ERROR” instead.
Multiply this negative number by the square footage to get the modified absorption for the Sabine equation. Lose the minus sign and calculate .05 x volume/modified absorption.
That’s right, the equation is:
Reverb time = .05 Volume/-1 x Surface Area x LN(1 - total absorption/surface area)
Now you can calculate the reverb time for most rooms. A good time for a small listening room is about one second. Art does have a say here and there are other things to worry about.
The Norris-Eyring equation is pretty good except when you put all your fuzz on one wall. The sound could bounce wall to wall and ceiling to floor all day long. That, of course, would be a resonance! If you do not have a rectangular room, you could talk about other kinds of resonators. Any space shaped like a drum or whistle? If you do, maybe there is a reason for those funny sounds!
You could use fuzz on every other surface to get rid of the resonances, but then your reverb time would probably be too short! Stay tuned for diffusion.
Absorption seems easy to understand; fluffy stuff absorbs sound. You sometimes ignore that the depth of the fluff determines how low in frequency the absorber works. When you ignore that, the resultant unbalanced reverb usually bites you hard enough in the ear to pay attention. You might prefer that the reverb time increases as the frequency decreases, but too much of it becomes muddy or muffled. Too much absorption and the music dies.
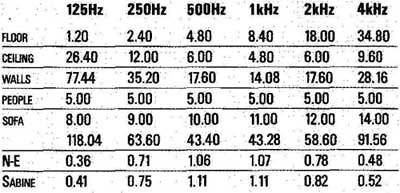
In your listening room, it is likely that you already have too much absorption. A 10’ x 12’ x 8’ drywall room with a thin carpet on the floor, a single sofa, and two people will have a 500Hz reverb time of:
Floor 10x12x.04=4.8
Ceiling 10x12x.05=6
Sidewalls 2x8x10x.05=8
Longwalls 2x8x12x.05=9.6
Person 2 x 2.5 = 5 [Typical number for humans]
Sofa 1 x 10=10 [Guess based on fuzzy area]
Total absorption = 43.4 Sabins
Volume 10 x 12 x 8 = 960ft^2
Surface area 2x10x12+2x8x 22=592ft^2
The Sabine reverb time would be:
.05 x 960/43.4 = 1.106 seconds
The N-E reverb time would be .05 x 960/-1 x 592 x LN(1 - 43.4/592) = 1.065 seconds
Of course, your room will probably have a door and maybe windows, which will lower the reverb time. A larger room will tolerate more absorption before it be comes too dry. That is why a bigger room is better for listening.
TAMING THE ROOM
If by some chance your room starts off with too much reverb, you can add absorption. You should calculate the reverb time at all frequencies before you start. In our example room the reverb time would be that shown in Table 2.
As you can see, the walls move enough to either absorb or let the low frequencies out. You do not require any more low frequency absorption. The high frequencies are also dead. If you could pick an absorber that just worked around 800Hz, you would be fine.
The first lesson here is that fuzz eats the high frequencies. The thicker the fuzz, the lower it works. Even lots of thin fuzz will not noticeably affect low frequencies, so it is quality not quantity that counts.
Lots of folks get this wrong and just keep adding thin fuzz and killing the midrange and highs and still have lousy bass. When fuzz cannot be thick enough, it is time for a diaphragm type of absorber. The standard fuzz we use is R25 8” thick x 24” wide unfaced fiberglass. We try to hide it behind panels or in special containers that also absorb low frequencies.
The second lesson is that walls flex and let Out low frequencies. The more solid the wall the less low frequency absorption/transmission and fewer problems with the neighbors. When taming a room by ear, start big and thick. Work on con trolling the low frequencies first, because what you do with them usually will also affect the high-frequency problems. If you start with thin material or high-frequency fixes, they will not affect the lows and you very likely will end up with a dead room that still has problems. First go low!
Diaphragms, such as a heavy drape, very thin walls, or anything that moves with the sound and is well damped, absorb the low-frequency energy (Lesson 2!). You could use fiberglass to back a set of thin walls; only the low frequencies would pass through the surface and become trapped. Or you might consider a special thin membrane of wood or stretched fabric.
Some special concrete blocks have a slit in them that allows energy into the inside of the block where it is then reduced by fiberglass stuffing. You can buy bass absorbers that match your needs, or you can build some. Remember: the larger the diaphragm, the lower the frequencies at which it stops working. If it is not well damped it will add a resonance to your room. This may or may not sound bad depending on how under-damped it is and at what frequency it occurs.
If you do not choose to change the appearance of your room, try hiding the fuzz. The underneath of a sofa is often hollow. If you put a bag of fizz here, only the high-frequency energy that bounces off the carpet would be absorbed. More of the midrange would get in and the very lows would pass through. A taut fabric hidden here would remove some of the low-frequency energy.
The more useful trick is to control the midrange without killing the high frequencies: Diaphragms for low frequencies, fuzz for highs, and a good balance to get the midrange right. You should already know to take the grille cloth off your loudspeakers for best results. If you think it is the fizz in the cloth that kills the highs, that is only part of the answer. Uniform holes act as filters. The holes’ size and spacing determine the frequencies of interest.
You can construct a baffle of a thin surface material with holes in it backed by fizz to absorb midrange energy. The high frequencies will penetrate. To a small degree most will be bounced back by the surface material. At just the right frequencies some will pass to the fizz, some will bounce, and some will become twisted in phase on the bounce and cancel out a bit. A well-designed absorber will have some diaphragm action for lows, holes for midrange, and fizz for highs.
 Table 3: You con use this worksheet to determine absorption.
Table 3: You con use this worksheet to determine absorption.
ABSORBER CONSTRUCTION
As an example, in churches we use an absorber that is 20” x 60” x 6” made from ¼ birch plywood, all painted black. There are 888 /i6” holes in the face of the box, and the back is open. It is stuffed with 8” of fiberglass compressed into 6”, and the face and sides are wrapped in a grille cloth. With a white grille cloth, we use a black fabric to cover the face to keep the holes invisible. These absorbers hang a few inches down from the ceiling, allowing them two chances at the sound energy.
You can make a simple corner absorber from fiberglass hardware cloth and fabric.
Purchase a galvanized steel wire mesh called hardware cloth or screen from your hardware supplier. This is woven from thin steel wire that has been hot-dip-galvanized and forms a mesh with ¼" openings. This is sold in 3 'widths and is avail able by the roll or cut to length. Six to 8' is usually enough to make one absorber.
I use 78" because that length fits nicely in my car. When I cut the hardware cloth, I wear leather gloves and use aircraft straight snips and try to cut in the center of the cell. Then I use needlenose pliers to bend the sharp edges back over the end wire and press down the sharp tips.
Use an un-faced R25 8” thick x 15” wide fiberglass. Put on your latex gloves and safety glasses. Cut two pieces almost the length of your hardware cloth. I use 72”. You can place this about 2” in from the long edge of the hardware cloth and roll it closed.
Try to use cable ties to hold it together until you solder the seam. Be sure the hardware cloth cells line up with each other or else your absorber will tilt when you are done. I count four cells in and two down to start with and cable-tie both ends. Then I add about six more ties care filly spaced and pull the seam almost together.
Solder the seam using a 40W iron and 60/40 rosin core .032” solder. The seam wires should just touch as you solder them. Apply just a drop of solder every 4” for about a 24” length. Then solder a bead from drop to drop. After it cools, fill the small gap and go on to the next section.
I just use black duct tape to attach the black fabric covering. Use a 36” wide cloth that is intended for gardening, but if you are using these in a public space you should use a fire-resistant cloth. You could also wrap the absorber with a nice-looking piece of fabric that matches your decor and sew the seam. Fold over the top and bottom and join them in the center.
You should place the absorber in a corner about 4” from each wall. The walls and spacing make it more effective at low frequencies.
Some people use a Helmholtz resonator to absorb specific frequencies. In one practical application it resembles a large flowerpot partially filled. Think of a small indoor tree in an over-large pot with a narrow tapered top one-third filled with dirt. Adjust the topsoil to tune it.
PLAYING THE ROOM
The best classic reverb time for speech intelligibility in a small room is about one-half of a second. Any less and too much of the speech is absorbed also. Any longer and the intelligibility scores begin to drop. The horrible news is that if you make the room optimum for speech, then it is too dead for music. The good news is that you do not need the maximum speech intelligibility in most cases. You just need good enough.
in a concert hail you can live with the high end of acceptable reverb times, because the music is more important. In a multipurpose hall, you can vary the absorption. That way when a play comes in, the room will be deader.
Speech intelligibility is at maximum once the signal-to-noise-ratio in a room reaches 20dB. This signal-to-noise might work for heavy metal, but the symphony crowd would write you very nasty notes.
A room that is really bad for speech also hinders music. Even though many organists yearn for very long reverb times to increase the power of the music, if it is not properly controlled, all the notes just smear together in a muddle. The definition of the music is lost.
The reverberant field theory that most folks work with assumes a clean uniform decay without sharp echoes or resonances that distort the decay of the energy in the room. A room’s reverb is called linear if the decay when plotted on graph paper is a straight line. The graph paper should have the vertical (Y) axis as a six decade log scale to represent the 60dB of decay. The horizontal (X) axis should be linear. When the plotted curve wiggles a bit, the room is nonlinear. This is often caused by the room’s geometry, materials, and construction.
I prefer multipurpose rooms that are nonlinear. A sharp drop of 10dB followed by a gradual rolloff can give a classic re verb time of several seconds for the music and still have excellent speech intelligibility.
A good room will allow you to hear the first sounding of a note and still support the music. That is why I prefer nonlinear rooms. The quick drop of 5-10dB in the reverb time curve will give detail to the music and allow speech intelligibility. The tail can extend for several seconds giving a longer classic reverb time. The exact drop depends on the primary use of the room.
For more modern music a reverb time of 2.5 to 3 seconds may be more appropriate. This is where Art sings volumes. You can judge when your adjustments to your room are right by your listening tests or measurements.
In the next installment (coming soon), we’ll look at the problems associated with reverb.
============