
Communication trade journals, micropower oscillators, thermodynamic basics, steam calliope sources, and avoiding energy scams.
By DON LANCASTER
Electronics cannot stand on its own. It has been built on the underlying foundations of math, physics, chemistry, and engineering disciplines.
This month, I figured I'd take a back-to-the-basics tack over a topic that has been causing more than its share of helpline grief lately. In short, we are long past overdue in taking a close look at the... Fundamentals of thermodynamics As Fig. 1 shows, a heat engine is some device or machine that accepts thermal energy at a high input temperature TH, performs some useful mechanical work, and finally loses its remaining to a sink at a lower temperature TL. Nearly all auto and airplane power sources are heat engines, as are most electrical generators. Variations on the heat-engine theme lead us to heat pumps, ice makers, vacuum systems, refrigerators, and compressors. And, for that matter, to life itself.
The study of heat processes is known as thermodynamics. While it is more of a mechanical engineering topic than an electrical one, electrical-engineering students are usually required to take at least one thermodynamic course. That's because nearly all modern heat engines need lots of support electronics, and many home-power and new energy-recovery schemes are actually heat engines in disguise.
Knowing the fundamentals of thermodynamics lets you instantly sort out the perpetual-motion rip-offs and the outrageous pipe dreams from the realities of physics and real-world economics.
We should note up front that more people have spent more time hacking heat-engine ideas than on any other engineering topic. The field has been studied to death, and there are tons of readily available literature out there.
Unless you can bring something truly new to the table, the chances are that your "new" heat-engine concept has been thoroughly trashed decades ago. Or even centuries before. For instance, I got this model heat engine in the mail. It was a clever example of how crucially important reversibility is in preventing you from getting useful economic benefits.
Reversibility is a very sticky thermodynamic problem that was first solved in a brilliant analytic breakthrough by James Watt in 1784.
Thermodynamic
Any study of thermodynamics starts off with the laws of thermodynamics. To the best of my knowledge, nobody has ever been able to find any exception to these laws. At least not on a practical scale.
Countless individuals have blown incredible amounts of their time and money trying to crack those thermodynamic laws. And all of them have failed miserably. Without any exception.
The laws are pretty fundamental, and they range well beyond heat engines. For instance, cell biology, the very life process itself, photosynthesis, all chemical reactions, and solar power all must rigorously obey these laws.
NEED HELP?
Phone or write your Hardware Hacker questions directly to: Don Lancaster Synergetics Box 809 Thatcher, AZ 85552 (602) 428-4073 Heat energy always travels from higher temperatures to lower ones.
Systems always move toward their most chaotic and lowest energy-level states. Energy can neither be created nor destroyed in any thermodynamic process.
If you do build a heat engine, only a small fraction of the total energy can ever be recovered as useful work; the rest is irretrievably lost as waste heat.
Ah yes, that fraction. How big and just how much? Enter one of the most brilliant hardware hackers of all time, a French scientist named Sadi Carnot. Like most hardware hackers, Carnot did not have the foggiest idea what he was really It took the establishment decades to understand how utterly profound and fundamental Carnot's discovery was.
Typical heat engines depend on a working fluid. The more important heat-engine fluids include air, steam, ammonia, freon, mercury, nitrogen, hydrogen, helium, and even liquid sodium. The working fluid goes through a number of individual steps or processes to trace out a cycle. A heat-engine cycle must close upon itself and return to initial conditions so that the engine can continue to produce useful work.
These thermodynamic cycles can usually be plotted in your choice of a pressure-volume 1 p-v) diagram or a temperature-entropy It-s) diagram.
The area inside the cycle on either a pressure-volume or temperature-entropy diagram is related to the amount of useful work that can be extracted from your heat engine.
The efficiency of any engine is the ratio of the useful work you extract compared to the energy that must be dumped as waste heat expressed as a percent.
Many different heat-engine cycles have been dreamed up. The Otto cycle applies to gasoline engines, while the Diesel cycle obviously applies to diesel power plants. Electric-power turbines use a Rankin cycle. Or some multi-stage improvement on it. A lot of alternate-energy people get real excited over a Sterling cycle, since it is a kind of "external combustion" engine that can accept heat from diverse or low-grade sources.
Every few years or so, somebody reinvents the old Stirling engine, and then builds a bunch of prototypes that just barely miss. Then they will just barely go bankrupt. To date, the old Stirling cycle has largely proven to be both a sucker bet and an economic rat-hole. Except possibly for arcane cryogenic cooling applications.
Carnot asked two questions: What is the finest theoretical thermodynamic cycle you could possibly create, and what are the efficiency limits to that cycle ?" It was obvious that each part of a Carnot cycle had to be lossless.
More precisely, all the processes involved had to be reversible. A heat engine must be able to undo anything it just did without losing any heat to friction or other losses. That means "no sudden moves" and "no rapid changes," with everything changing as incrementally and as slowly as possible. That also means zero friction, lossless seals, and perfect insulation. Figure 2 helps to explain the concept of reversibility.
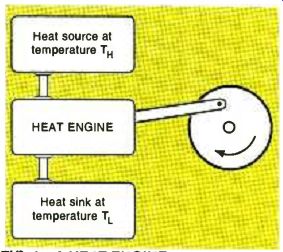
FIG. 1--A HEAT ENGINE accepts energy from a high-temperature source,
does useful mechanical work, and then rejects a portion of that
energy to a lower-temperature sink. Only a fraction of the available
energy can ever be converted. Most electronic devices must also
obey the same thermodynamic laws that govern heat engines.
Suppose you want to use a piston to compress the gas inside a non-insulated cylinder. Some work is needed to reduce the gas to a required volume. Any work above that is lost as waste or exhaust heat.
Let's say you build up your cylinder and throw an eight pound weight on it. You note that this particular piston happens to drop by a foot, doing work to compress the gas. In this case, eight foot pounds of work are expended, because the eight-pound weight dropped by one foot.
Is this the best you can do? If instead, you put a one-pound weight on the piston, waited until things settled down, added another one-pound weight, and so on, you now have the first pound pushing the piston a full foot and the final pound pushing the piston down only an eighth of a foot. Your total work is 8/8 + 7/8 + 6/8 + 5/8 + 4/8 + 3/8 + 2/8 + 1/8 = 4.5 foot-pounds.
Yet, in each case, you compressed the gas by the same amount, raising its energy state by the same value. The extra 3.5 foot-pounds of work has dropped through that irrecoverable low-grade heat rat hole.
In both examples, you have an irreversible process. But your second one is clearly much better. As a third try, put a feather on the piston.
Wait till things settle, and then put another feather on the piston. Repeat this until you have accumulated eight pounds of feathers, and you will also end up with the gas compressed by a foot. And only 4.0 foot-pounds of total work will be needed.
This is a reversible process and the best you can do. And twice as efficient as your first attempt.
One more time: You must be able to undo what you just did. At all times, you do have to be willing and able to stuff the Genie back into the bottle. That's what reversibility is all about.
There are several important reversible processes. One of them is called an isothermal process, where everything takes place at a constant temperature. The heat-energy receiver must be at a temperature only a tad lower than the energy source. And the heat source must be so large that taking a small amount of energy out of that source does not significantly change its temperature.
A second one is an adiabatic process, in which heat energy is neither added nor removed. For full reversibility, any compression or expansion of a working fluid should be adiabatic.
At any rate, the Carnot cycle obeys fully reversible processes to trace out the best approximation of the theoretically ideal heat-engine cycles. Figure 3 shows some more Carnot cycle details. Once again, this is the best possible one you can ever build. Why? Because of the full reversibility. You just can't get better than this.
In the Carnot cycle, one starts with a working fluid at a low temperature. Then the gas is adiabatically compressed by doing work on it.
Typically, the new external energy required can come from a flywheel or a second cylinder in the engine.
Compressing the fluid raises its temperature and reduces its volume. Extreme care is needed so that heat energy is neither added nor removed during compression.
The temperature rise has to result solely from the volume reduction.
Compression will continue until a temperature very slightly under that of the heat source is reached. After compression, an isothermal process transfers its heat energy into the fluid, expanding it, and increasing its pressure. The temperature, of course, stays the same. In the third step, the gas adiabatically expands while delivering useful output work. The fluid volume goes up and the temperature goes down. A final isothermal process dumps the remaining heat energy to the waste heat sink, reducing the volume and closing the cycle.
Once again, that area inside the curve on the pressure-volume diagram determines how much work is delivered.
Because every process is reversible, one can introduce heat energy at a high temperature to produce mechanical work output. Or, one can apply mechanical work to pump heat from the low side to the high side.
A gas engine runs "forward." A refrigerator runs "backward." And a heat pump can either heat or cool, by swapping the heat from the high-side to the low-side.
When you go through the math (the derivation takes a full lecture hour in any university physics course), this stunningly elegant and simple result pops out ... eta = (TH TL) /TH Restated in English: The very best possible efficiency that can be obtained from any heat engine is determined solely by the ratio of its source temperature to its sink temperature-no matter what cycle, fluid, or engineering is used! And my key point: To get useful work out of any heat engine, lots more energy must be thrown away as irrecoverable waste heat.
For example, a modern auto engine has a theoretical efficiency of 55 percent, with something like 38 percent being a typical real-world value. That is at the engine's flywheel. The actual efficiency of the automobile as a transportation system is far lower.
Oh yeah. One minor gotcha. Absolute temperatures must be used for the Carnot formula to work. Absolute degrees Rankin are 460 degrees above Fahrenheit. Absolute degrees Kelvin are 273 degrees above Centigrade. And any efficiency is a hundred times the efficiency stated as a fraction from 0.0 to 1.0. Down my street is an impressive artesian hot well that's spewing forth mightily at 125 degrees F. Could we get some electricity out of it? Assume the local average ground temperature is 75 degrees (The well sits in the Upper Sonoran life zone.) The best possible energy recovery efficiency here would be...
((125 + 460) (75 + 460))/ (125 + 460) = 0.085
Or a mere 8.5 percent, under ideal conditions. That's assuming a perfect Carnot cycle. In the real world, you'd be lucky to get a tiny fraction of that-say three percent net. For every kilowatt hour generated, thirty-two are thrown away! Sadly, it makes sense to ignore some $20,000 worth of electricity each year just by dumping it into the Gila River. Why? Because any practical recovery project of this size and temperature differential could never pay for the engineering, materials, operating costs, flood zone risks, and the time value of money needed to do the job properly.
--------------
As one process in a heat engine cycle, assume you have a fluid you wish to compress by adding weight to the piston such that your piston drops by exactly one foot ... On your first try, you throw an eight pound brick on the piston and note that the piston does in fact drop by one foot. You have done 8.0 foot pounds of work. As we will shortly see, this is a useless and BADLY IRREVERSIBLE process, because one-half of your work will get lost as irrecoverable low grade heat... On your second try, you place a one pound rock on the piston, wait till things settle, and then add another one pound rock. You will still need eight pounds of rocks, but this time, the work needed is only 8/8 + 7/8 + 6/8 + 5/8 + 4/8 + 3/8 + 2/8 + 1/8 = 4.5 foot pounds of work. This is a useful but MODERATELY IRREVERSIBLE process in which one-eighth of your work gets thrown away... On your third try, you place a feather on the piston, wait till things settle, and add another feather. You still need eight pounds of feathers, but this time the work needed is only the tiniest amount above 4 foot pounds. This is a FULLY REVERSIBLE process in which virtually none of your work gets thrown away... Any fully reversible scheme to compress or expand a fluid is also called an ADIABATIC process. Heat is neither added nor removed in any reversible adiabatic process.
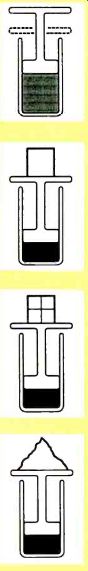
FIG. 2--AN EFFICIENT HEAT ENGINE absolutely demands that all of
its processes be fully reversible. Here are some good and bad examples
of reversibility.
---------------
The AREA inside the Carnot cycle determines how much useful mechanical work is output.
The EFFICIENCY of a Carnot cycle is determined solely by this elegantly simple formula:
n = (TH-TO/TH = OT/TH
You can multiply this 0-1 value by 100 to get the percentage efficiency. Note that an ABSOLUTE temperature scale must be used such as Kelvin (Centigrade + 270) or Rankin (Fahrenheit + 460). Note that the best possible efficiency of any heat engine depends ONLY upon the high-side /low-side temperature differential and nothing else!
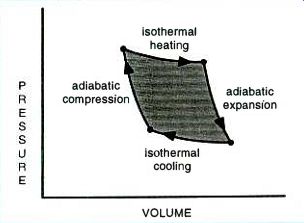
FIG. 3--THE MOST EFFICIENT HEAT ENGINE you can possibly build conforms
to the Carnot Cycle. All four processes are fully reversible. Even
the best Carnot cycles must dump a lot of non-recoverable energy
as waste heat.
--------------
Is there any way you can beat the thermodynamic laws? Generating electricity won't hack it, because always use your electricity to run a motor, which instantly gets you back to a heat engine which has to rigorously obey the laws.
You can switch to a binary or even a trinary cycle which uses multiple stages and different fluids. But these often will only marginally improve the efficiency while doubling or even tripling the costs.
The only other way I know of is to do something useful with the rejected waste heat. Say by heating water, equalizing process temperatures, or starting your own catfish farm.
A solar panel that also heats water should have much better economics than one that solely generates electricity. Schemes such as these are sometimes called cogeneration, and they are now a very hot topic indeed. There's even a World Cogeneration trade journal.
A reality check
So, how do you separate the "real" heat engines from the scams and the wishful thinking daydreams? First, calculate its Car-not efficiency and see what that reveals about the claims involved. Be especially suspect of statements like "this is not a heat engine," or the thermodynamic laws do not apply to me." Then carefully look at the p-v or t-s diagrams (these will always be very obvious on any legitimate device). Then check carefully for anything that is obviously nonreversible. Any sudden changes or the need to add coolant to close a cycle are both dead giveaways that all is not well. Always ask Can each and every process be run backward just as well as forward ?" Then throw in some economic analysis. This could be your time to break even. That's the length of time needed for generated power to pay for the materials, the design, and time value of the money needed to create the device. Assume that 17.58 watts is a heating rate of one BTU per minute, and that it raises one pound of water by one degree F. And that a nickel per kilowatt-hour is a sensible ballpark cost for electricity that you can avoid. And that a gallon of water weighs eight pounds. And that the time value of money normally triples the cost of a project.
Note that the time to break even can easily be infinite! If you produce less revenue from electrical generation per year than the interest on the construction financing, the longer you run, the more you will lose.
Finally, be extremely wary of the word " Stirling." When and where it happens to occur.
My favorite popular thermodynamic book is Heat Engines by Sanford. It is found in the Double-day Science Series. Beyond that one, pick up any of the dozens of college-level course texts. Important thermodynamic resources include the SAE Library from the Society of Automotive Engineers, and the EPR/ Publications from the Electric Power Research Institute.
Of the many trade publications, Power Engineering is typical; it targets large-scale electrical generation. And, of course, virtually any and all thermodynamic papers ever published are instantly available through Dialog.
Communications resources
Where can you go to get a crystal for a pager? Or to find out more on fiber-optic communication? Or information on wireless modems? Or phone testing? Or computer local area networks? As always, your first and foremost source of insider information in any field is in the trade journals.
As our resource sidebar for this month, I have gathered together a bunch of the more obscure and more fragmented communications trade journals for you. I have a hunch I might have missed a few here, so be sure to let me know if you have any other favorites.
Crystal oscillator
Hacking a crystal oscillator is no big deal these days. You just bias any old CMOS gate into its active region and hang a crystal in the feedback path. But things get tricky fast if you try to run at ultra-low supply currents or want to start oscillation reliably at unusually low voltages.
Most hackers have discovered that typical CMOS transistors run out of gain at ultra-low currents. And they ultimately find that the best route to micropower oscillators is to use bipolar transistors instead of CMOS gates.
Harris Semiconductor has just introduced a cheap and very simple HA7210 all-CMOS crystal-oscillator chip. It costs under a dollar and free samples are available. As Fig. 4 shows, you simply hang a crystal on the chip, and away you go.
The current consumed by this oscillator is a mere five microamps at 32 kilohertz.
Pin 6 is an enable; keep it high to run and make it low to disable the chip. Pins 7 and 8 can program the operating current. Make them both high for low frequencies (10-100 kHz) and both low for high frequencies (5-10 MHz). The supply-voltage range for the chip is 2.0 to 7.0 volts.
New tech lit
There are some new integrated circuits for this month: The SSI 67F687 is a new engine interface peripheral offered by Silicon Systems. It receives gasoline-engine crank and cam input signals for use as timing and pollution management.
From Trident, a new Video View video-processing chip set offers scalable, full-motion video windows and frame capture for VGA systems. From Hitachi, there's a new HD49049FS picture-in-picture controller chip, also intended for video-insertion applications. The Grass Valley Group has a fine video Dictionary of Technical Terms newly available. A good bookstore for navigation texts and GPS satellite secrets is the Navitech Book & Software Store.
One source for Apple II repairs and supplies is Rolf Taylor. The best Apple II magazine is still
Resource
+ 2 to +7 vdc output square wave

FIG. 4-THIS MICROPOWER CRYSTAL OSCILLATOR from Harris Semiconductor
needs only five microamps of supply current from a source as low
as two volts. It costs under a dollar. Free sample kits are available.
Central. And the A2 GEnie Round-Table has just seen its 20,000th library uploads. Yet another trade publication for this month is the Industrial Laser Review. A good source for steam calliopes is Ragtime. It offers a $5 catalog.
Free samples of Volara, Volextra, and Minicel foams are available from Voltek. Ask for their specifier kit.
A reminder here that I have autographed copies of my Incredible Secret Money Machine ll here for you at my own Synergetics. This book is a must if you are starting up your own tech venture. Plus a reminder about my Hardware Hacker Round-Table on GEnie PSRT at (800) 638-9636. And our no-charge technical helpline is found at (602) 428-4073. Best calling times are 8-5 weekdays, Mountain Standard time.
Most of the items I've mentioned appear in our Names & Numbers or Communications Trade Journals sidebars. Be sure to check here first before calling our helpline. Let's hear from you.
Also see: DRAWING BOARD